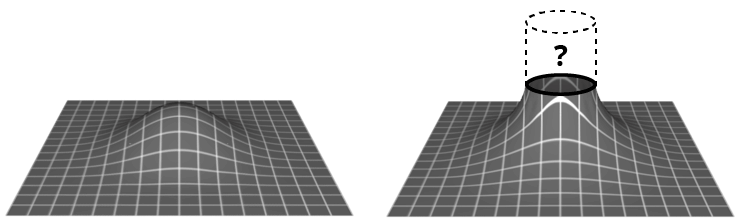
The inside of a black hole is hidden behind broken equations and a gravitational field so strong that even light cannot escape. Although general relativity predicts that black holes exist, the theory breaks down at the event horizon. It is widely believed that understanding the inside of a black hole will require a new theory of physics: a theory of quantum gravity.

General relativity uses spacetime geometry to explain gravity. Knot Physics extends this geometric description by assuming that spacetime is a branched manifold. In Knot Physics, both quantum mechanics and gravity result from the behavior of the branches of spacetime. This theory of quantum gravity makes predictions about the interior of black holes, extending our knowledge of physics beyond the event horizon.

The Breakdown of General Relativity

---

Before considering Knot Physics, we explore general relativity’s predictions and limitations.

General relativity posits that the presence of matter results in spacetime curvature and that this curvature is gravity. The theory prescribes a relationship between the distribution of mass and the curvature that it produces, but, in so doing, general relativity also predicts its own failure: Past a certain density of matter, the curvature of spacetime produces a black hole, and the theory cannot predict the curvature of spacetime or the distribution of matter inside of that black hole.

We can understand some aspects of curvature by considering the geometry of a circle. We can imagine a circle on spacetime and two radial lines on the interior of the circle. In the absence of curvature, there is a well-known relationship between a circle’s radius r and its circumference \( c,\) which is \(c=2 \pi r\) or equivalently \(r = c/2 \pi.\) In this case, the radial lines will meet each other at the center of the circle at a distance \(r = c/2 \pi\) from the circle’s circumference.

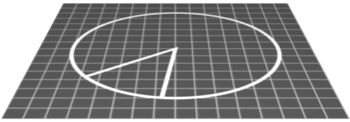
The presence of matter results in spacetime curvature. This curvature implies that the circle’s radius has increased relative to its circumference. As such, the circle’s radial lines span a distance longer than \(c/2 \pi\) before converging at the center of the circle. In addition to curvature of space, this spacetime curvature also implies time dilation: a clock near the center of the circle will advance more slowly than a clock that is far away.

Past a certain density of matter, general relativity predicts an amount of curvature that effectively rips spacetime. The length of the radial lines goes to infinity, so the radial lines never converge. This is a black hole. A clock that is falling toward the black hole experiences a time dilation so intense that, to an outside observer, the clock appears to take an infinite amount of time to reach the event horizon. At the event horizon, general relativity can no longer predict the curvature of spacetime or the distribution of matter.

The “ripping” of spacetime that general relativity predicts inside a black hole is commonly perceived to signal the breakdown of the theory. Another instance where general relativity breaks down is quantum mechanics. It is widely believed that understanding black holes will require a theory of quantum gravity.

The “ripping” of spacetime that general relativity predicts inside a black hole is commonly perceived to signal the breakdown of the theory.

In Knot Physics, we extend the geometric reasoning of general relativity to create a geometric model of quantum mechanics. We will show how that geometric model of quantum mechanics naturally produces gravity. We then go on to show how this theory of quantum gravity reveals the physics inside black holes.

A Geometric Model of Quantum Mechanics

---

Knot Physics uses a geometric model of spacetime to explain quantum mechanics.

In Knot Physics, the 4-dimensional spacetime manifold is embedded in a 6-dimensional space.

The spacetime manifold is a branched manifold consisting of a large, but finite, number of branches. The branches of spacetime can split and recombine.

Fermions—like electrons and quarks—are knots in the spacetime manifold.^[1] One fermion consists of one knot on every branch of spacetime. Each knot can move around on its own branch, independently of the knots on other branches. Each branch is a state in a quantum superposition.

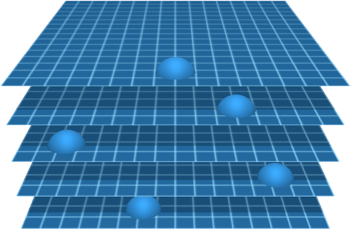
The geometry of each knot can be described with a complex amplitude which we call the quantum amplitude. The sum of the quantum amplitudes of the knots on all branches is the quantum wave function for the fermion.

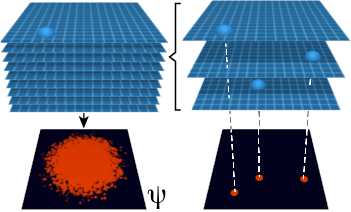
When branches recombine, their knots can recombine. The complex quantum amplitudes of the knots are additive at recombination. This addition of quantum amplitudes is quantum interference.

Knot Physics assumes that spacetime is a branched manifold, and quantum mechanics results from the behavior of those branches. The branched spacetime manifold also results in gravity.

Knot Physics assumes that spacetime is a branched manifold, and quantum mechanics results from the behavior of those branches.

Quantum Gravity

---

We showed how quantum properties result from the properties of the branched spacetime manifold. We now show how entropy maximization of the branched spacetime manifold produces gravity.

Curvature and Entropy

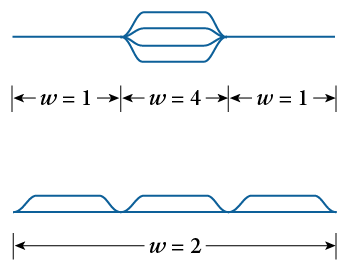
Curvature is related to entropy through the behavior of the branches of spacetime. The branches of spacetime can split and recombine. This branch recombination obeys certain rules. We assume there is a branch weight \(w\) that is defined at every point on the spacetime manifold and that \(w≥ 1\) everywhere. We also assume that branch weight is conserved at branching. As such, a branch with branch weight \(w\) can split into at most \(w\) branches.

The spacetime manifold can also stretch. Total branch weight is conserved when the manifold stretches. As such, stretching the spacetime manifold causes the branch weight to distribute over a larger region.

These rules imply a relationship between branching and stretching. Stretching causes the branch weight to distribute over a larger region, which reduces the average branch weight on the manifold. This reduction in branch weight implies that the manifold cannot branch as many times.

Subject to these rules, the branches of the spacetime manifold randomly split and recombine. This branch recombination has entropy. Entropy of branch recombination is greater when branches recombine more frequently. Entropy of branch recombination can therefore be impaired by stretching the spacetime manifold: Stretching reduces the number of possible branches, which reduces random recombination of branches. Reduced branch recombination implies reduced entropy.

We now relate this entropy to curvature. In Knot Physics, the 4-dimensional spacetime manifold is embedded in a 6-dimensional space, and curvature is literally the bending of the spacetime manifold inside that larger space. (By contrast, in general relativity, there is no “outside” of spacetime. General relativity defines the curvature of spacetime in terms of a metric that does not have any additional context.) Increasing the scalar curvature of spacetime implies stretching the spacetime manifold. Because stretching impairs entropy, scalar curvature impairs entropy.

Matter and Entropy

Aside from curvature, the entropy of the spacetime manifold is also related to other factors. The next factor we consider is matter.

Matter impairs the entropy of the spacetime manifold in several different ways. Branch recombination has entropy, and entropy of branch recombination is greater when branches recombine more frequently. A fermion consists of one knot on every branch of spacetime, and the branches of spacetime can only recombine if the knots on those branches match. For that reason, the presence of knots on the spacetime manifold is an obstruction to branch recombination. This reduction in branch recombination impairs entropy.^[2]

Matter also impairs the entropy of spacetime in other ways. Some of these forms of entropy impairment correspond to fundamental forces like electromagnetism.^[3]

Branch Weight Distribution and Entropy

In addition to curvature and matter, the entropy of the spacetime manifold is also related to branch weight distribution.

Branch weight is a conserved quantity. The entropic process of diffusion tends to cause branch weight to spread to a uniform distribution. Likewise, nonuniform distribution of branch weight impairs entropy.

Gravity from Entropy

The spacetime manifold maximizes entropy by minimizing the entropy impairment of curvature, matter, and branch weight distribution. The result is gravity.

The spacetime manifold maximizes entropy by minimizing the entropy impairment of curvature, matter, and branch weight distribution. The result is gravity.

Matter impairs entropy. The entropy impairment of matter can be reduced by decreasing the 4-dimensional volume of spacetime where entropy is impaired.^[4] That 4-dimensional volume takes into account not only spatial dimensions but also duration in time.

The 4-dimensional spacetime manifold is embedded in a 6-dimensional space. Motion of the matter in those 2 additional spatial dimensions causes time dilation.^[5] In other words, a clock on the spacetime manifold near the matter would advance more slowly than a clock on the spacetime manifold far away from the matter. This time dilation decreases the 4-dimensional volume of spacetime in the region around the matter. The decrease in volume reduces the entropy impairment of the matter.

Motion of the matter in the 2 additional dimensions causes waves in the spacetime manifold. These waves result in curvature. Although the curvature impairs entropy, the total entropy impairment of both matter and curvature is minimized.

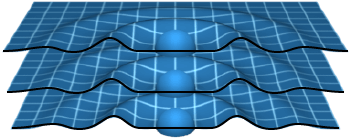
Earlier, we established that entropy maximization implies that branch weight tends to be uniformly distributed. When branch weight is uniformly distributed, the spacetime curvature caused by these waves matches the curvature described by general relativity.^[6] In other words, when branch weight is uniformly distributed, Knot Physics and general relativity make equivalent predictions about gravity.

When branch weight is uniformly distributed, Knot Physics and general relativity make equivalent predictions about gravity.

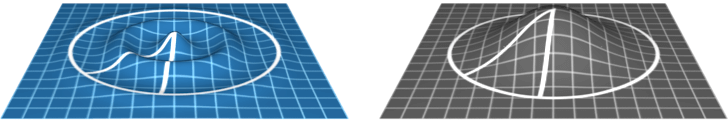
However, while typically entropically disfavored, nonuniform branch weight distribution is also possible. When this is the case, Knot Physics and general relativity make different predictions about spacetime curvature. Such is the case with black holes.

Black Holes

---

In Knot Physics, gravity of a massive object allows for two possible equilibria. In the first equilibrium, branch weight is uniformly distributed, and the geometry of spacetime matches the description of general relativity, as described above. In the second equilibrium, branch weight is not uniformly distributed, and the geometry of spacetime diverges from general relativity’s predictions. In this case, the massive object is in a highly compressed state: a black hole.

There are two quantum effects that typically obstruct the compression of matter. The first obstruction is the Heisenberg uncertainty principle. In Knot Physics, a fermion consists of one knot on each branch of spacetime. Those knots move independently of each other, each on its own branch. The Heisenberg uncertainty principle as applied to Knot Physics states that the distribution of a fermion’s knots on the branches of spacetime has some inevitable variance. This variance implies that the fermion’s knots cannot be perfectly coincident with each other; rather, they must be distributed over some volume of spacetime. The second quantum effect that obstructs the compression of matter is the Pauli exclusion principle, which states that two identical fermions cannot occupy the same state. Together, the Heisenberg uncertainty and Pauli exclusion principles limit the compression of matter.

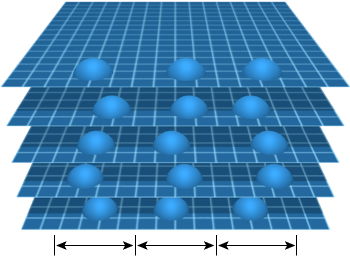
Exceeding this limitation on the compression of matter requires eliminating quantum mechanics as a constraint. Quantum mechanics results from the interaction of the branches of spacetime. If there is only one branch of spacetime, there is no quantum behavior. Because a fermion consists of one knot on each branch of spacetime, a fermion on one branch consists of just one knot. In this case, the Heisenberg uncertainty principle no longer holds: The fermion can be contained within a space as small as the volume of an individual knot. If there are multiple fermions on that single branch of spacetime, the individual knots that are the fermions can be immediately adjacent to each other.

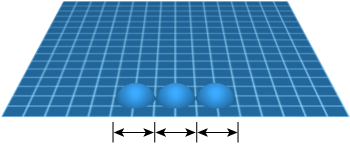
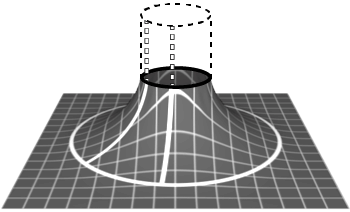
With quantum mechanics eliminated as a constraint, the fermions of the massive object are compressed to individual knots immediately adjacent to each other on a single branch of spacetime. Additional compression results from the size of each knot being minimized. Fundamental principles of Knot Physics imply that the topology of a knot requires a gradient of branch weight.^[8] For an isolated knot, this gradient implies that branch weight close to the knot is higher than branch weight further from the knot. Fundamental principles also imply that reducing the size of a knot requires increasing the gradient of branch weight. Increasing the gradient of branch weight requires nonuniform branch weight distribution. Nonuniform branch weight distribution impairs entropy. The entropy impairment can be minimized with a particular configuration of knots wherein all of the knots collectively share a single, large gradient of branch weight. In this configuration, the knots are arranged in a thin spherical shell that is just one knot thick, where the interior of the shell contains no knots. The branch weight of the empty spacetime manifold on the shell’s interior reduces to its minimum possible value, \(w = 1,\) while the branch weight on the exterior of the shell remains high. This difference of branch weight maximizes the gradient of branch weight across the shell of knots. As a result, the size of each knot in the shell is minimized.^[9]

Matter is now in its most compressed state: a shell of particles on a single branch of spacetime where each fermion is compressed to the width of a single knot and each knot is compressed to its minimum possible size. This state is a black hole. The shell of matter is located at the event horizon.^[10] The interior of the black hole is a single empty branch of spacetime.

This state is a black hole. The shell of compressed matter is located at the event horizon. The interior of the black hole is a single empty branch of spacetime.

To reduce the entropy impairment of matter, the shell of matter at the event horizon moves in the 2 additional spatial dimensions of the 6-dimensional space in which the spacetime manifold is embedded. This movement causes tremendous time dilation near the black hole. The resulting waves imply curvature, which is the gravity of the black hole.

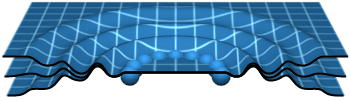
Outside the black hole, the curvature of spacetime caused by these waves matches the curvature of spacetime described by general relativity. But inside the black hole—where general relativity ceases to describe spacetime curvature—Knot Physics predicts that spacetime is flat.

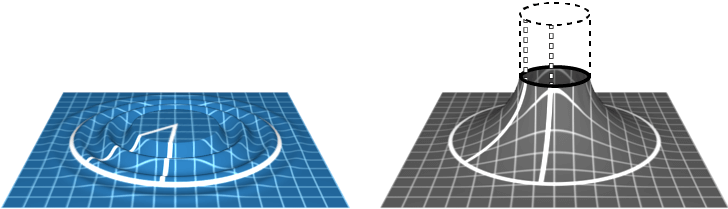
Matter and energy can fall into the shell of matter at the event horizon, but, once they are part of that shell, the entropy of the spacetime manifold is an obstacle to their escape. At the event horizon, there is only a single branch of spacetime. Away from the event horizon, that single branch of spacetime splits into many branches. Since a fermion consists of one knot on each branch of spacetime, a fermion escaping the event horizon would have to transition from being a single knot on one branch to being many knots on many branches. In so doing, the fermion would transition from impairing entropy on just one branch of spacetime to impairing entropy on many branches of spacetime. This is strongly entropically disfavored and is therefore a significant obstacle to fermions escaping the black hole.

Matter and energy can fall into the shell of matter at the event horizon, but, once they are part of that shell, the entropy of the spacetime manifold is an obstacle to their escape.

The same principle extends to other particles, including photons. Every real particle consists of one instance on each branch of spacetime. Escaping the black hole would require that a particle transition from impairing entropy on one branch to impairing entropy on many branches, which is strongly entropically disfavored.

While extremely unlikely, escape from the event horizon is possible. This possible occurrence is Hawking radiation.

---

Outside a black hole, physics obeys the familiar rules of quantum mechanics and general relativity. The branches of spacetime allow quantum behavior, while maximization of entropy results in a relationship between matter and curvature that matches general relativity.

Drawing near to a black hole, time dilation and gravity increase, as general relativity predicts. At the event horizon, all the matter that has fallen into the black hole forms a thin shell on a single branch of spacetime. Here, the laws of quantum mechanics cease to apply. Particles in the shell at the event horizon are packed in beyond the limits typically imposed by quantum mechanics and compressed to their minimum size. Entropy makes escape from the event horizon extremely unlikely.

Past the event horizon, there is no matter, no gravity, and no quantum mechanics: just a single, empty branch of spacetime.

In Knot Physics, the branched spacetime manifold maximizes entropy, and this maximization of entropy governs the dynamics of both gravity and quantum mechanics. At the most extreme behavior of the spacetime manifold, the branched structure collapses, and spacetime forms a black hole.

Notes

---

1. For more on fermions, see Theory Summary: Fermions.
2. For more on how matter impairs entropy through reduction of branch recombination, see Schrödinger’s Cat.
3. For more on how matter impairs entropy through fundamental forces, see Theory Summary.
4. Combining the entropy impairment of matter and curvature we get \(w(L _m + R).\) The entropy impairment of matter and curvature in a region A of spacetime is therefore \(S = \int_{A} w(L_m + R) \,dM.\) Branch weight is a conserved quantity, which implies that maximizing entropy is equivalent to minimizing this entropy impairment. In the case of gravity, minimizing this impairment occurs with a spacetime geometry that includes time dilation. The time dilation reduces the volume measure \(dM\) at the location of impaired entropy and, in this way, it minimizes the entropy impairment.
5. The 6-dimensional space in which the spacetime manifold is embedded is a Minkowski 6-space. Motion in a Minkowski space results in time dilation.
6. Note that these waves are standing waves that correspond to a static gravitational field. These waves are not “gravitational waves” in the sense typically used in general relativity.
7. Where branch weight distribution is nonuniform, Knot Physics makes predictions for spacetime curvature that are different than general relativity. Branch weight is nonuniform in several cases. The first case is black holes. The second case allows an explanation of dark matter: Over large distances, the distribution of branch weight may be nonuniform, and this variation of branch weight may explain astronomical effects that are attributed to dark matter without the need for additional particles. (For more on dark matter, see the paper Knot Physics: Dark Matter ⇗.) The third case occurs close to a particle: Fundamental principles of Knot Physics imply that the topology of a particle requires a gradient of branch weight, which implies that branch weight is not uniformly distributed. This discrepancy is consistent with other conflicts between general relativity and quantum mechanics close to a particle. (For more information about fermion topology and branch weight gradient, see the paper Physics on a Branched Knotted Spacetime Manifold ⇗.)
8. For more information about fermion topology and branch weight gradient, see the paper Physics on a Branched Knotted Spacetime Manifold ⇗.
9. The interior of the shell of knots is empty in order to maximize the branch weight gradient across the shell. A particle requires a branch weight gradient. If a particle were on the interior of the black hole, its branch weight gradient would increase branch weight on the interior of the black hole such that \(w > 1.\) This would decrease the difference in branch weight between the interior of the black hole and the exterior, thereby reducing the branch weight gradient across the shell of knots. This reduction in branch weight gradient would increase the size of the knots at the event horizon, reducing the compression of matter in that shell. For this reason, optimal compression of matter in a black hole occurs when the interior of the black hole has no knots.
10. The Bekenstein bound is an upper limit on the amount of information that can be contained within a finite region of space with a finite amount of energy. This bound is saturated by the entropy of black holes as calculated by the Bekenstein-Hawking boundary entropy. This Bekenstein-Hawking entropy is proportional to the area of the event horizon. In Knot Physics, the matter that falls into a black hole forms a shell that is one particle thick at the event horizon. As such, the amount of matter in the black hole is proportional to the area of the event horizon. This allows a comparison between the information in the matter and the area that it covers at the event horizon of a black hole.

Learn More

---