At the center of physics is a conundrum that has persisted since the early days of quantum mechanics.

Schrödinger’s famous thought experiment involves a cat in a box. The cat is locked in a box with a vial of poison gas. The gas will be released if a radioactive atom decays. From outside the box, the experimenter does not know whether the cat is alive or dead. Schrödinger’s thought experiment illuminates a perplexing question: How can we reconcile quantum and classical physics?

Schrödinger’s thought experiment illuminates a perplexing question: How can we reconcile quantum and classical physics?

The radioactive atom is a small quantum system. By the rules of quantum mechanics, the nucleus of the atom can be in a superposition of multiple states. Perhaps it is even reasonable to think of the atom as being in a superposition of decayed and not yet decayed. Then maybe the vial of gas can be in a superposition of released and not yet released. Maybe even the cat can be in a superposition of dead and alive. The atom, the vial, and the cat all consist of particles that obey quantum rules. Is it reasonable to say that there is no transition between quantum and classical descriptions, and even the largest configurations of particles can exist in a quantum superposition of all possibilities?

In Knot Physics, the answer to that question is no. The reason is the relationship between quantum mechanics and entropy.

Quantum Mechanics in Knot Physics

---

We will describe a few aspects of quantum mechanics in Knot Physics that will help us unravel the quantum conundrum of Schrödinger’s cat. (For more on quantum mechanics, see Theory Summary: Quantum Mechanics.)

Branches in Spacetime

In Knot Physics, spacetime is a branched manifold consisting of a large, but finite, number of branches. The branches of spacetime split and recombine randomly.

Fermions—like electrons and quarks—are knots in the spacetime manifold. One fermion has one knot on every branch of the spacetime manifold. Each knot can move around on its own branch, independently of the knots on other branches.

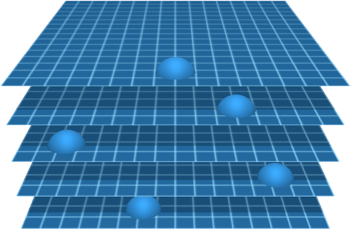
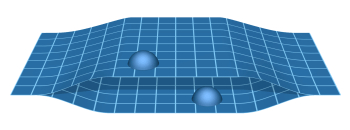
In order for two branches to recombine, the knots on each of the recombining branches must match.

The branches cannot recombine at any location where the knots do not match. (Branches can still recombine if both branches have no knots.)

Branches and Quantum Mechanics

In Knot Physics, we use the branched spacetime manifold to explain quantum mechanics. We also use the branched spacetime manifold to explain the limits of quantum mechanics. Quantum mechanics makes correct predictions as long as certain constraints are satisfied. Those constraints arise from the entropy of branch recombination.

In Knot Physics, we use the branched spacetime manifold to explain quantum mechanics. We also use the branched spacetime manifold to explain the limits of quantum mechanics.

The branches of spacetime split and recombine randomly. That branch recombination has entropy, and the branched manifold will tend to maximize that entropy. The entropy of branch recombination is greatest when the branches recombine frequently, and the branches recombine most frequently when the knots on the branches frequently match.^[1] As such, entropy favors distributions of knots that frequently match.

Suppose we begin with some initial distribution of knots (corresponding to one or more particles). We can use quantum mechanics to attempt to predict how that distribution of knots will change over time. If quantum mechanics predicts a knot distribution such that the knots frequently match, then the predicted knot distribution does not impair the entropy of branch recombination. In that case, quantum mechanics can successfully predict the distribution of knots.

For example, quantum mechanics could successfully predict the distribution of knots for an electron in an atomic orbital. Quantum mechanics is successful because the knots of the electron frequently match and therefore do not impair the entropy of branch recombination.

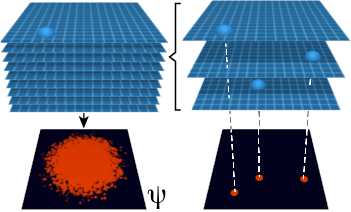
However, quantum mechanics makes incorrect predictions in certain circumstances. This is the case with Schrödinger’s cat.

The Cat

---

A short aside: Schrödinger’s cat example is more dramatic than we need. Rather than a vial of poison gas, we will assume instead that the box contains a container with a cat toy inside. If the radioactive atom decays, then the cat toy will be released.

In this thought experiment, quantum mechanics would predict that—like the nucleus of the radioactive atom—the cat toy could also be in a superposition. For the cat toy, that would be a superposition of both contained and released.

Let’s consider the implications of such a superposition from the Knot Physics perspective. The knots of the cat toy in a superposition of both contained and released would occupy a large region of spacetime. In that region, the cat toy knots would not match across different branches. As a result, the branches would not be able to recombine in that large region, which would cause a large impairment to the entropy of branch recombination.

In Knot Physics, a superposition of this size can never occur. Entropy maximization causes the knots to converge to a similar arrangement rather than differ from each other in a large region. While quantum mechanics would predict a large superposition, branch recombination entropy prevents it. The cat toy will either be released on all branches or contained on all branches.

While quantum mechanics would predict a large superposition, branch recombination entropy prevents it.

---

By assuming that spacetime is a branched manifold, we can have our quantum cake and eat it too. For systems that do not impair branch recombination, the behavior of knots is well-described by quantum mechanics. For systems that do impair branch recombination, the entropy of branch recombination forces the system to obey classical rules.

Notes

---

1. We can state the “frequent matching” constraint more precisely. The distribution of knots also has entropy, in addition to the entropy of branch recombination. The spacetime manifold maximizes the total entropy, which results in an equal amount of action \(ℏ\) in each degree of freedom. “Frequent matching” means that each degree of freedom in the distribution of knots does not impair the branch recombination entropy by more than \(ℏ.\) Similarly, there can be no measurement that constrains the distribution of knots any more precisely than that limit (from which we can obtain the Heisenberg uncertainty principle).

Learn More

---